Jan 28, 2017: Constructing Kites and Conjectures

• ← Previous
• All
• Next →

Ms Van Donselaar presented on 2.3, giving a construction of a kite. We talked a little about how to write that up, and then had a long conversation around this particular item.

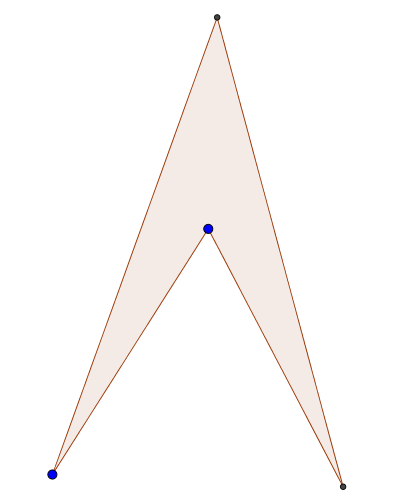
One particularly interesting feature of the construction that Ms Van Donselaar pointed out was that her method really produces two kites with a given side AB. One of them looks like we expect. The other is “odd-looking”, in Mr Peter’s words, and seems to have the property that its diagonals don’t cross. If that can be tightened up, we might get a resolution of Conjecture 2.2. We decided to call such things “boomerangs.”

We generated a list of new questions to add to our work:

Question E: Is it possible to construct a kite which has no equilateral triangle? (The Van Donselaar examples all have triangle ABC as an equilateral triangle.)

Question F: What is an “interior angle?”

Question G: What is the “interior” of a polygon?

Question H: How do we differentiate between the “normal-looking” and the “odd-looking” kites? Is there some geometrical way of distinguishing these?

At the end of the hour, we had several people waiting: Carpenter with 2.5, Lewis with 1.5, and Maass with 2.1.